Visit: www.ravindrawaykolesciphy.blogspot.com
For 11th and 12th Students
USE OF LOGARITHMIC TABLES & OTHER MATHEMATICAL TABLES
In Physics practicals and numerical problems we are required to use the logarithmic tables for calculations.
The logarithmic tables are used for multiplications, divisions, squaring and finding the roots of the numbers. Logarithm of a number consists of the following two parts:
(i) Characteristics (C):- Integral part
(ii) Mantissa:- Decimal part.
logarithm of a number = Characteristic + Mantissa
For example : log 49 = 1.6902
In the answer before decimal number 1 is the Characteristics and .6902 is mantissa.
(A) Determination of the Characteristics:–
(i) Characteristics for numbers greater than or equal to 1:
The Characteristics of such number is positive.
Rule: Count the number of digits (d) in the integral part of the given number, subtract 1 from it and you will get the characteristics (C).
i.e. C = (d-1).
Some examples:
(ii) Characteristics of a number less than 1:
Such numbers have only decimal part. The characteristics of such number is negative.
Rule: Count the number of zeros (z) immediately following the decimal part; add 1 to it and you will get the characteristics i.e. C and is read as bar C.
Examples:-
(B) Determination of Mantissa: Rule: To find the mantissa, first convert the given number in four figure number by approximation. For example: 273.16 ⇒ 273.2
Divide given four figure number into three groups as:
Refer the logarithmic table and search two digit number i.e. 27 given in the first box in the first column the of the Logarithmic table.
Consider the row with the number 27. In this row, the number under the column headed by Third digit i.e. 3 is 4362.
In the same row, the number in the Mean Difference column headed by 2 is 3.
Add this number 3 to 4362 gives 4365. The mantissa for the logarithm of the given number is 0.4366. The characteristics of the number 273.2 is 2.
Therefore the
log 273.2 = 2 + 0.4365 = 2.4365
Part –B: Antilogarithm (Abbreviation: Antilog)
It is the reverse process of logarithm that is you are given the logarithm of a number in the form ⇒
log N = Characteristics + Mantissa
i.e. log 273.2 = 2.4365
From 2.4365, we have to find original number N (i.e. 273.2).
∴ Antilog (log N) = N
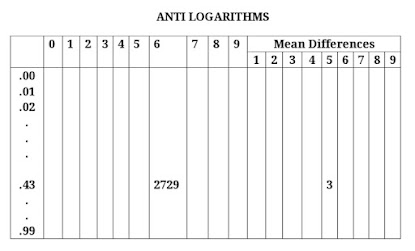
Rule: Consider the four figure mantissa part in the decimal part (with the decimal point) of 2.4365 (i.e. .4365)
Now refer the Antilogarithmc Table, use the same method as you use for finding the logarithm.
Now add add number 3 to 2729 gives as 2729+3 = 2732
Use the following rules, to find the number of digits in the integral part of the number. i.e. Position of Decimal point in the final answer.
RULES: (1) If the characteristic is zero the number of digit in the integral part of the number is one.
(2) If the characteristic is positive, the number of digits in the integral part of the number is one more than the characteristics. (i.e. C + 1).
(3) if the characteristic is negative the number of zeros following the decimal point
( before the first significant figure) is one less than the numeral value of the characteristics.
For Example: If the characteristic is 2, put the decimal point after first three digits. If the characteristic is bar 3, write two zeros between decimal point and first digit.
∴ Antilog (2.4365) = 273.2 OR 2.732 × 10²
LOG AND ANTILOG OF SOME NUMBERS
Laws (Theorems)of Logarithms
PART:C
Use of Reciprocal Table
Take the first for digits of one digit- integer number. Find the first two digit number with decimal point between in the first column of the reciprocal table. Follow the same procedure as for logarithm of number, but the number from the main difference column has to be subtracted.
For Example: Reciprocal of 3.372 = 1/ 3.372, find 3.3 in the first column; proceed along in row to the column headed 7 the figure there is 2967 then we proceed along the same row to the mean difference part and find the figure in the column headed 2.
It is 2. Subtract it from 2967 and we get 2965.
∴ 1/ 3.372 = 0.2965
Rules: 1) Reciprocal of a number more than one:
Experess the given number in the form of First two digit number with decimal point between multiplied by a factor of appropriate + ve power of 10 and find the reciprocal.
Example: 1/751.6 = 1/ 7.516× 10² = 0.1331 × 10-³ = 0.001331
2) Reciprocal of a number less than 1:
Experess the given number in the form of First two digit number with decimal point between multiplied by a factor of appropriate - ve power of 10 and find the reciprocal.
Example: 1/ 0.03216= 1/ 3.216× 10-² = 0.3109× 10² = 31.09


EXERCISE-2:
Find the reciprocals of :
(i) 73.5 (ii) 591.1 (iii) 0.4216 (iv) 0.08192 (v) 0.003572
Use Trigonometric Tables, Natural Sines, Natural Cosines Natural Tangents Tables.
Note: The method of using the table is similar to that for logarithm of a number.
For sine & tangent table, add the mean difference but for cosine table subtract the mean difference.
1) Find sin θ for following the angles :
(a) 30⁰ (b) 60⁰ 36' (c) 24⁰ 15'
2) Find cos θ for following the angles :
(a) 40⁰ (b) 54⁰ 12' (c) 23⁰ 25'
3) Find tan θ for following the angles :
(a) 45⁰ (b) 39⁰ 24' (c) 50⁰ 7'
EXERCISE-4 Inverse of trigonometric values:
Find the angle θ in the following cases:
(i) sin θ = 0.1739 (ii) cos θ = 0.9390
(iii) tan θ = 1.2550
## ANSWERS:
EXERCISE-1
1) 3526 2) 15.81 3) 67.08
4) 13.81 5) 76.96 6) 25.01 7) 3.004 8) 0.001541
9) 12.92 10) 16.21
11) 1.984 × 10⁶ 12) 5.001×10¹² 13) 3.005 14) 5.961
EXERCISE-2
(i) 0.136 ×10 -¹ = 0.01361
(ii) 0.1692 × 10 -² = 0.001692 (iii) 0.2378 × 10 ¹ = 2.378 (iv) 0.1221 × 10 ² = 12.21 (v) 0.2803 × 10 ³ = 280.3
EXERCISE-3
1) (a) 0.5000 (b) 0.8712 (c) 0.4107
2) (a) 0.7660 (b) 0.5850 (c) 0.7179 3) (a) 1.0000 (b) 0.8214 (c) 1.1967
EXERCISE-4
(i) θ = 10⁰ 1' (ii) θ = 20⁰ 7' (ii) θ = 51⁰ 27 '
*******END*******

.png)










.jpg)